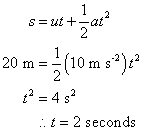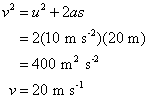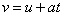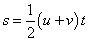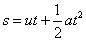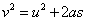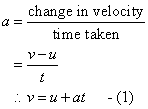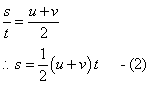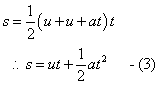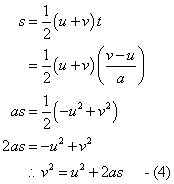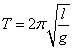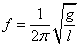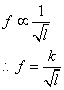Archimedes' principle is a law that explains buoyancy or upthrust. It states that
When a body is completely or partially immersed in a fluid it experiences an upthrust, or an apparent loss in weight, which is equal to the weight of fluid displaced.
According to a tale, Archimedes discovered this law while taking a bath. After making this discovery, he is said to have leapt out of his bathtub and ran through the streets of Syracuse naked shouting "Eureka!".
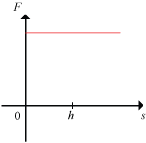
An object experiences upthrust due to the fact that the pressure exerted by a fluid on the lower surface of a body being greater than that on the top surface, since pressure increses with depth. Pressure,
p is given by
p =
hρg, where:
h is the height of the fluid column
ρ (rho) is the density of the fluid
g is the acceleration due to gravity
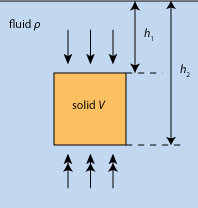
Let us confirm this principle theoretically. On the figure on the left, a solid block is immersed completely in a fluid with density
ρ. The difference in the force exerted,
d on the top and bottom surfaces with area
a is due to the difference in pressure, given by
d =
h2aρg –
h1aρg = (
h2 –
h1)
aρgBut (
h2–
h1) is the height of the wooden block. So, (
h2 –
h1)
a is the volume of the solid block,
V.
d =
Vρg∴ Upthrust =
VρgIn any situation, the volume of fluid displaced (or the volume of the object submerged) is considered to calculate upthrust, because (
h2 –
h1) is the height of the solid block only when it is completely immersed. Furthermore, the pressure difference of the fluid acts only on the immersed part of an object.
Now, moving back to
Vρg. Since
V is the volume of fluid displaced, then the product of
V,
ρ and
g is the weight of the fluid displaced. So, we can say that
Upthrust = Weight of the fluid displaced
Compare this conclusion with the statement above summarising Archimedes' principle. Are they the same? Well, not totally. The “apparent loss in weight” was not mentioned in my explanation.
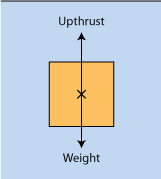
In the figure on the left, there are arrows on the top and bottom of the solid block. The downward arrow represent the weight of the block pulling it downwards and the upward arrow represent the upthrust pushing it upwards. If one were to measure the weight of the solid block when it is immersed in the fluid, he will find that the weight of the block is less than that in air. There is a so-called “apparent loss in weight”, because the buoyant force has supported some of the block’s weight.
| Weight in air – Upthrust | = | Weight in fluid |
| Upthrust | = | Weight in air - Weight in fluid |
∴ Upthrust = Apparent loss in weight
Objects Floating FreelyWhen an object is floating freely (i.e. neither sinking nor moving vertically upwards), then the upthrust must be fully supporting the object’s weight. We can say
Upthrust on body = Weight of floating body
By Archimedes’ principle,
Upthrust on body = Weight of fluid displaced
Therefore,
Weight of floating body = Weight of fluid displaced
This result, sometimes called the “principle of floatation”, is a special case of Archimedes’ principle and can be stated:
A floating body displaces its own weight of fluid.
If a body cannot do this, even when completely immersed, it sinks.
See Also
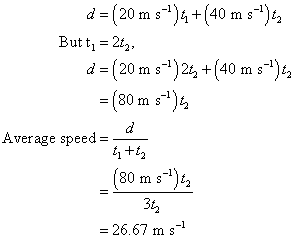



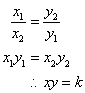
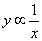
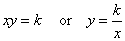
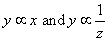
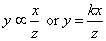

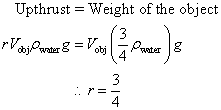
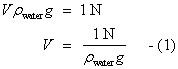
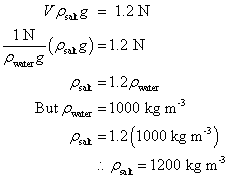
 Let us confirm this principle theoretically. On the figure on the left, a solid block is immersed completely in a fluid with density ρ. The difference in the force exerted, d on the top and bottom surfaces with area a is due to the difference in pressure, given by
Let us confirm this principle theoretically. On the figure on the left, a solid block is immersed completely in a fluid with density ρ. The difference in the force exerted, d on the top and bottom surfaces with area a is due to the difference in pressure, given by In the figure on the left, there are arrows on the top and bottom of the solid block. The downward arrow represent the weight of the block pulling it downwards and the upward arrow represent the upthrust pushing it upwards. If one were to measure the weight of the solid block when it is immersed in the fluid, he will find that the weight of the block is less than that in air. There is a so-called “apparent loss in weight”, because the buoyant force has supported some of the block’s weight.
In the figure on the left, there are arrows on the top and bottom of the solid block. The downward arrow represent the weight of the block pulling it downwards and the upward arrow represent the upthrust pushing it upwards. If one were to measure the weight of the solid block when it is immersed in the fluid, he will find that the weight of the block is less than that in air. There is a so-called “apparent loss in weight”, because the buoyant force has supported some of the block’s weight.