The Relationship between Variables (Part II)

This article is a continuation from Part I, which discusses about direct proportion.

Inverse Proportion

Two sets of readings for the quantities x and y are given in the table below.

x	3	4	6	12
y	4	3	2	1

Here, when x is doubled, y is halved; when x is trebled, y is one-third its previous value and so on.

We say that y is inversely proportional to x, or y varies inversely as x, that is

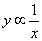
The graph of y versus x is as shown:

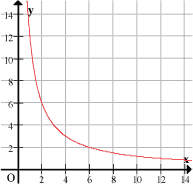
Notice that the product of x and y is the same for every point (12 in this case). Hence this product is known as the constant of proportionality or variation and it is given a symbol such as k. Therefore, we can write the exact relationship between x and y as

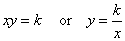
Assuming that there are points (x₁, y₁), (x₂, y₂), (x₃, y₃), …, we can say

xy = x₁y₁ = x₂y₂ = x₃y₃ … = k

Notes:

1. When a variable is proportional to two or more variables, we can represent this relationship as a joint variation. For example,
   When
   y ∝ x and y ∝ z
   therefore
   y ∝ xz or y = kxz
   
   When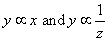
   
   therefore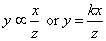
   

2. In every proportional relationship, the converse is also true. For example,

If y ∝ x, then x ∝ y.

Examples of physical quantities that vary inversely with each other are:

• Pressure and volume
• Frequency and periodic time
• Gravitational force and the distance squared

To be continued…